Lecture 1t (PDF)

A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering

MN50429 - Financial Engineering

Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Lecture 1

A partial review: Black-Scholes and beyond
Dr Andreas Krause and Dr XiaoHua Chen

The Black-Scholes option pricing model: Main
assumptions and formula
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

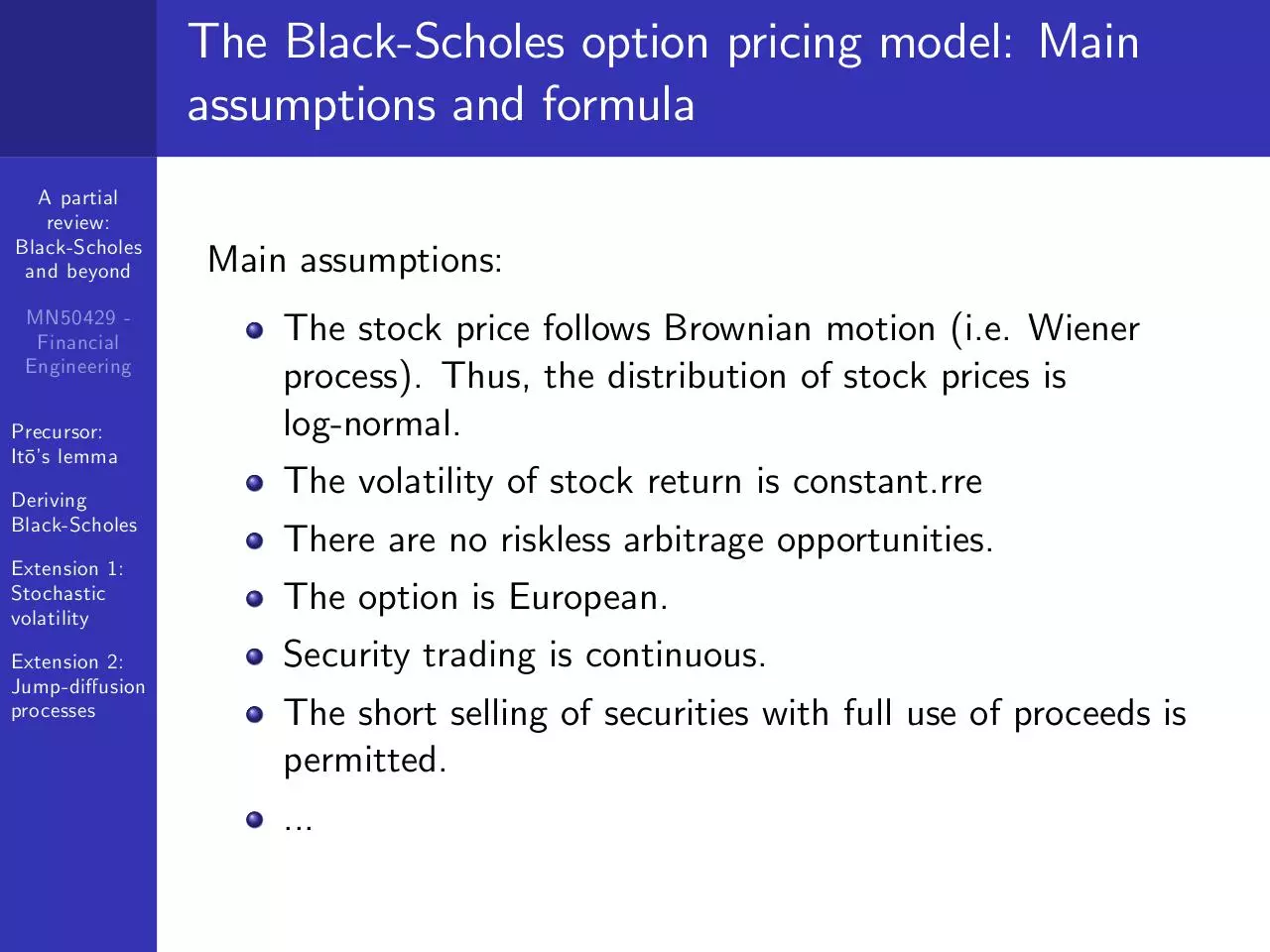
Main assumptions:
The stock price follows Brownian motion (i.e. Wiener
process). Thus, the distribution of stock prices is
log-normal.
The volatility of stock return is constant.rre
There are no riskless arbitrage opportunities.
The option is European.
Security trading is continuous.
The short selling of securities with full use of proceeds is
permitted.
...

The Black-Scholes option pricing formula
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

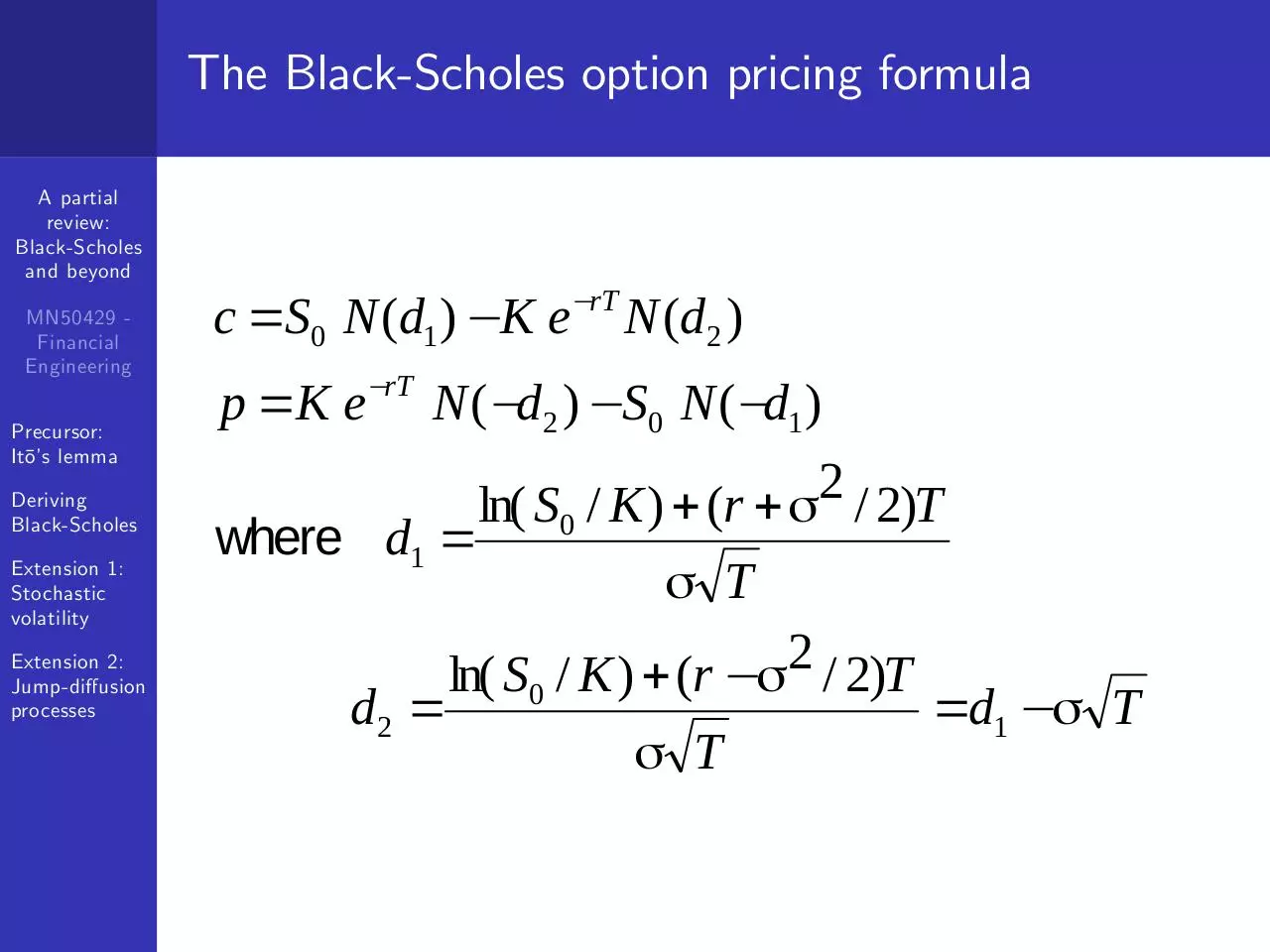
c = S0 N (d1 ) − K e−rT N (d2 )
p = K e−rT N (−d 2 ) − S0 N (−d1 )
ln( S0 / K ) + (r + σ2 / 2)T
where d1 =
σ T
ln( S0 / K ) + (r − σ2 / 2)T
d2 =
= d1 − σ T
σ T

The need to go beyond Black-Scholes
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma

The market consists of a wide variety of derivatives

Deriving
Black-Scholes

Payo¤ structures are di¤erent

Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Underlying assets’stochastic processes are di¤erent

Need for accurate pricing
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Issuers need to be able to price derivatives in order to sell
them appropriately
Hedgers need to be able to price derivatives in order to
obtain cost-e¢ cient solutions to their risk exposures
Traders need to be able to price derivatives in order to
ensure an e¢ cient market

A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma

1

Precursor: It¯o’s lemma

2

Deriving Black-Scholes

3

Extension 1: Stochastic volatility

4

Extension 2: Jump-di¤usion processes

Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Brownian motion
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

A common assumption is that stock returns are normally
distributed
St St
St

For

t
t

ln St

ln St

t

N

t;

2
t

t ! 0 we can write the dynamics as
dSt
= dt + dzt
St

(1)

is the drift (expected return)
is the volatility
p
dZt = "t dt is a Wiener process, " N(0; 1). Wiener
process is a partricular type of Markov stochastic process
with a mean change of 0 and a variance rate of 1.

A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Source: Hull, J, Options, futures, and other derivatives, 7th ed., p.264.

Functions of stochastic processes
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

The value of a derivative will be a function of the value of
the underlying asset and time
Second order Taylor series approximation:
C (St ; t)

C (St

t; t

t) +

@C
(St
@S

@C
(t (t
t))
@t
1 @2C
+
(St St t )2
2 @S 2
1 @2C
+
(t (t
t))2
2 @t 2
@2C
+
(St St t ) (t (t
@S@t

St

t)

+

t)) (2)

A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Note: second order Taylor series approximation formula:
f (x) = f (x0 + h) = f (x0 ) + f 0 (x0 )h + 12 f 00 (x0 )h2

It¯o’s lemma
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

In Eq.(2), If we move C (St
t ! 0, this becomes
dC

=

t; t

t) to LHS and, let

@C
1 @2C
@C
dS +
dt +
(dS)2
@S
@t
2 @S 2
@2C
1 @2C
2
(dt)
+
dSdt
+
2 @t 2
@S@t
@C
@C
1 @2C
dS +
dt +
(dS)2
@S
@t
2 @S 2

This relationship is knows as It¯o’s lemma

(3)

Application to Brownian motion
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes

From Eq.(1), we have
p
dS = Sdt + Sdz = Sdt + S dt"
As dt ! 0 and E ["2 ] = 1, we have
(dS)2 =

2 2

S (dt)2 + 2

=

2 2

) dC =
+ S

2 2

S dt"2

S dt

Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

3

S 2 (dt) 2 " +

S

@C
dz
@S

(4)
@C
1
+
@S
2

2 2@

S

2C

@S 2

+

@C
@t

dt
(5)

This is the basis from which to derive the Black-Scholes
formula

A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma

1

Precursor: It¯o’s lemma

2

Deriving Black-Scholes

3

Extension 1: Stochastic volatility

4

Extension 2: Jump-di¤usion processes

Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Hedge portfolio
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

De…ne a portfolio V of the option C and a short position
of units of S, i.e. the underlying asset
V =C

S

) dV = dC

(6)
dS

(7)

Eliminating risk
Using Eqs. (1) and (5), the above portfolio of Eq.(7)
becomes
1 2 2 @2C
@C
@C
+
S
+
S dt
dV =
S
2
@S
2
@S
@t
@C
+ S
dz
(8)
@S
Eliminate risk by setting
) dV =

=

@C
@S

@C
1
+
@t
2

2 2@

S

2C

@S 2

dt

(9)

Riskless portfolio
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering

This portfolio is riskless, hence dV = rVdt, where r is the
risk-free asset rate of return.
Inserting Eq.(9) in the above and substituting V we have

Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

rv =

@C
1
+
@t
2

2 2@

S

2C

(10)

@S 2

Using Eq.(6), delta hedge = @C
@S and Eq.(10) we get the
Black-Scholes partial di¤erential equation:
@C
1
+
@t
2

2 2@

S

2C

@S 2

+ rS

@C
@S

rC = 0

(11)

Black-Scholes partial di¤erential equation
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

This equation holds for all derivatives where the
underlying follows a Brownian motion
Solution for a derivative depends on boundary conditions
Because a solution of a PDE is generally not unique,
additional conditions must generally be speci…ed on the
boundary of the region where the solution is de…ned.
Boundary conditions are typically values at expiry, values
at low or high prices of the underlying, etc.
Partial di¤erential equations can in general not be solved
analytically
Many can be solved numerically

European call option
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

For a European call option the boundary conditions are:
C (0; t) = 0
limS !1 C (S; t) = S
C (S; T ) = maxfS

E ; 0g

Solving for the Black-Scholes formula
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

With these boundary conditions exceptionally an analytical
solution exists
Derivation via variable substitution and Heat equation
using Fourier transforms (See Hull, J, Options, futures,
and other derivatives, 7th ed., p.307)
In future lectures we will look at numerical procedures to
solve such equations as well as other approaches to
determine the value of derivatives

A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma

1

Precursor: It¯o’s lemma

2

Deriving Black-Scholes

3

Extension 1: Stochastic volatility

4

Extension 2: Jump-di¤usion processes

Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Changing volatility
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Volatility is the main drive of the option value in
Black-Scholes
Volatility is not constant over time in real markets
More realistic to assume that it changes by some
mechanism, e.g. stochastic di¤erential equations (SDEs):
dS = Sdt +
d

2

= dt +

t SdzS

(12)

dz

(13)

dzS dz = dt

(14)

where is the volatility of , is a function of (S; 2 ; t).
is the correlation between the two stochastic processes.

Multivariate It¯o’s lemma
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering

The multivariate version of It¯o’s lemma is
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

dC

=

@C
@C
@C
dS +
d 2+
dt
2
@S
@
@t
1 @2C
1 @2C
2
+
(dS)
+
d
2 @S 2
2@ 4

(15)
2 2

+

@C
dSd
@S@ 2

2

Preliminaries
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

In Eq.(4) we derive
(dS)2 =

2 2

S dt
p
From Eq.(13), let dt ! 0, dz = "t dt, we have
d

2 2

=

2

=

2 2
t dt

dSd

2

3
2
2
t t (dt)

(dt)2 + 2

=
=

2 2 2
t t dt

(16)
3

S (dt)2 +
+

+

tS

t

S (dt) 2

(dt) 2 +

2
t Sdt

2
t Sdt

3

(17)

A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Plug Eqs.(4,16,17) in Eq.(15), we have
dC

@C
@t

=
+

+

1
2

2 S 2 @2 C + 1 2 2 @2 C
@S 2 2 2
@ 4
2S @ C
+
@S @ 2

@C
dS
|@S{z }

Uncertainty

+
1

@C
d 2
2
@
| {z }

Uncertainty

!

dt
(18)

2

Hedge portfolio
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

We have two uncertainties, so we need two instruments to
hedge
One instrument is the underlying asset S, the other is
b on the same asset S.
another option C
V =C

) dV = dC

b follows the same rules as C
C

S

dS

bC
b

b dC
b

(19)
(20)

Riskless portfolio
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Plug Eq.(18) in Eq.(20), we have
dV

=

@C
@t

b

@C
+
@S
|

!

2 S 2 @2 C + 1 2 2 @2 C
@S 2 2 2
@ 4
dt
2S @ C
+
2
@S @
!
b
b
b
@C
1 2 2 @2 C
1 2 2 @2 C
+
+
S
@t
2
2
@S 2
@ 4
dt
b
2 S @2 C
+
@S @ 2

+

1
2

b
b @C
@S
{z

Uncertainty

!

1

dS +
}

|

@C
@ 2

b
b @C
@ 2
{z

Uncertainty

(21)

!
2

d

2

}

A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering

To elimiate dS terms, Delta hedge:
@C
@S

Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

To elimiate d

2

b
b @C
@S

=0

(22)

terms, Vega hedge:
@C
@ 2

b
b @C = 0
@ 2

(23)

First step towards a solution
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma

Inserting the hedges and noting that dV = rVdt, plug
Eq.(19, 22, 23) in Eq.(21), we have:
@C
@t

+

=

b
@C
@t

Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

1
2

+

2 S 2 @2 C
@S 2

1
2

+

b
2 S 2 @2 C
@S 2

+

2 S @ 2 C + 1 2 2 @ 2 C + rS @C
rC
2
@S
@S @ 2
@ 4
@C
@ 2
2b
b
b
2 S @2 C
+ 12 2 2 @@ C4 + rS @@SC
@S @ 2
b
@C
@ 2

(24)

To hold Eq.(24), both side have to be equal to certain
function. We deduce the following function (ignore the
reasoning here):

b
rC

Linear structure
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

f (S;
where

2

and

; t) =

(S;

2

; t) +

(S;

2

; t)

(25)

are de…nded in Eq.(13)

and will in general be non-linear. Since they depend
on other variables so it’s not necessarily linear.
Combining the RHS and this function gives us a solution
to the di¤erential equation

Interpretation of
A partial
review:
Black-Scholes
and beyond

Assume we have a delta hedged portfolio:

MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

V =C

@C
S
@S

(26)

and applying It¯o’s Lemma in Eq.(18), we have:
dV

@C
@t

=
+

+

@C
d
@ 2

1
2

2 S 2 @2 C +
@S 2
2
+ 21 2 2 @@ C4

2

This portfolio still has volatility risk!

2 S @2 C
@S @ 2

!

dt
(27)

A partial
review:
Black-Scholes
and beyond

To elimiate the volatility risk, we plug Eq.(26) in the
following expression:

MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma

dV

rVdt = dV

(28)

Plug Eqs.(13, 25, 27) in the above Eq.(28), we have

Deriving
Black-Scholes
Extension 1:
Stochastic
volatility

@C
S)dt
@S

r (C

dV

rVdt = (
+

Extension 2:
Jump-di¤usion
processes

=

+

)

@C
dt
@ 2

@C
( dt +
@ 2 0

@C @
(S;
@ 2

dz )
2

; t)dt +

dz
|{z}

Uncertainty

1

A
(29)

Market price of volatility risk
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma

This expression dV
risk free rate

Deriving
Black-Scholes

Taking expectations, the last term dz vanishes

Extension 1:
Stochastic
volatility

The excess return (premium) increases in

Extension 2:
Jump-di¤usion
processes

rVdt is the excess return over the

is the market price of volatility risk

at a rate of

A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma

1

Precursor: It¯o’s lemma

2

Deriving Black-Scholes

3

Extension 1: Stochastic volatility

4

Extension 2: Jump-di¤usion processes

Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Jumps in stock prices
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Sometimes stock prices move by discrete amounts
Reasons can be crashes but also news arrivals, e.g
unexpected changes to earning, merger announcements,
etc.
Such discrete movements are called jumps

A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Representing jumps
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

dS
S

=(

m) dt + JdP( ) + dz

is the intensity, i.e. probability of observing a jump in
one time period
J is the size of the jump, which has some distribution with
m = E [J]
P( ) is a Poisson process
f (x) =

x

e
x!

;

> 0; E (x) = var (x) =

Other representations of jump-di¤usion processes exist

Known jump size
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

If we know the jump size we have two sources of risk: dz
and dP( )
As with stochastic volatility we proceed using a hedge
portfolio with two assets
Di¤erent di¤erential equation as stochastic process is
di¤erent

Unknown jump size
A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

This is not a third uncertainty
Uncertainty is about jump of size J1 , J2 , J3 ,....
For real valued jumps an in…nite number of uncertainties
exists
Market is then not complete
Derivative would then be a bene…t to the market, not a
mere replication of existing assets
It could not be perfectly priced, only price bounds be
determined

A partial
review:
Black-Scholes
and beyond
MN50429 Financial
Engineering
Precursor:
It¯o ’s lemma
Deriving
Black-Scholes
Extension 1:
Stochastic
volatility
Extension 2:
Jump-di¤usion
processes

Summary: The general steps to price derivative C
Use It¯o’s Lemma to derive dC
Construct riskless hedge portfolio V
Derive dV in the terms of stochastic process
Derive PDE by elimiating the risk in dV expression
through hedging
Solve the PDE either analytically or numerically. If
solution is numerical, set the boundary conditions for the
PDE and then solve.